General Report on Tunny
23G Page 91
itself at a different setting; for example, the extreme case of a "perfect" wheel such as
 |
(b) Rival settings.
At characters where two settings of a wheel agree, each setting will gain the same contribution for its ΔD count: if one of them is the right setting then at these characters the wrong ("slide") setting will have a systematically good ΔD count, elsewhere a systematically bad count. If the agreements are sufficiently numerous, this slide setting will have a score almost as large as the right setting, and may by random chance have a higher score.
With perfect wheels it is in fact, often difficult to distinguish between the right setting and its slides.
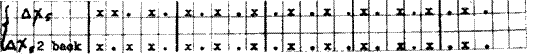
(c) Length of slides.
Owing to the absence of long stretches of dots or crosses in ΔΧ wheels, slides at interval 1 do not occur: a slide at interval 2 is by far the most common: it tends to produce consequent slides of 4, 6, ...
(d) Antislides.
In a run such as 1+2, which is unaffected by interchanging dot and cross in both ΔΧ1 and ΔΧ2, a good score will be obtained at settings with an excess of disagreements with the correct settings. This circumstance is called an antislide on both wheels. An antislide is usually at interval 1, and may be mistaken for a message slide until spanning is done.
(e) Setting slidy wheels.
When setting messages on wheels known to have strong slides, the most rapid method is to accept, provisionally, the highest score for a slidy wheel, even though there are others almost equally good, for on this basis it will generally be possible to set the other wheels. When all wheels are "set" at settings which are either correct or good slides, all the evidence of the 32 letter count will be available to discriminate between a correct setting and its slides. The evidence From, say, /, 5, U, may be adequate to set Χ1 uniquely, when the evidence of 1+2. is not.
Back to General Report on Tunny. Contents.