General Report on Tunny
24X Page 137
that the method would not be very powerful. Soon after this the square-summing test was suggested, emerging from some calculations which appear in the black file. These calculations contain an error (corrected below) but the order of the answer was right and agreed with the indications of the message just mentioned. It was not until September 1944 that the slide and significance test was invented (R3 pp 77, 83). It was not realised absolutely at once that this test is equivalent to the square-summing test. The original object of the slide and significance test was for putting rectangles in a priority order and even for rejecting them. Unfortunately the tapes took some time to make and the earlier Robinsons were rather hard on long tapes, so the rectangle was often converged before the sigma-age of the test had been worked out. It was suggested further that the slight modification of the test could be used for attempting to detect slides of ±1 (R3 p 92). This was tried only a few times and would probably have had an occasional success. The slide and significance test was made more practicable by the introduction of 'Thurlow tapes of the second kind' as the standard non-Colossus method of producing a rectangle (R4 pp 71, 82). However, the Robinson routine was dropped when the Colossus gadget, which counts the frequencies of occurrence of the different values of θij was brought in.
For another test for unconverged rectangles see R1 p 36. This test in effect is equivalent to a crude form of flagging a skeleton. Significance tests for flags are suggested in R2 p 92, and R3 p 8, and these can be regarded as tests for a rectangle on which no convergence has been done. But these tests would not be expected to do very well unless the rectangle is an exceptionally good one. On p 92, R2 there is also a suggestion which is a test rather of the start of a convergence.
An entirely different way of possibly obtaining evidence about the wheels without rectangling is by doing a Δ2Z alphabetical count (R3 p 64). This can be of value only if at least one of the Χ's has good Δ2 properties, i.e. Δ2 Χi nearly all crosses. (See also 25E(a) for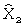 
runs and chapter 25F for one wheel break-ins if ab ≠ ½.)
(c) Tests for converged rectangles (historical).
The first rectangle ever done for wheel-breaking purposes is mentioned in Part 4. The first 10,000 letters of a message were
Back to General Report on Tunny. Contents.