General Report on Tunny
24F Page 129
24G SOME GENERALISED RECTANGLES
In order that the entry in each cell of a rectangle shall be a single number only a single condition can be imposed on the two impulses involved. The condition must therefore be of the form i + j /(known ΔD) =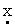 
, with or without fixed conditions.
Among the plain i + j rectangles 4+5, 2+5, 1+3, 3+4x, 2+4 have all been tried: indeed at one time it was erroneously supposed that 4+5 would be better than 1+2 (see 24Y(a)).
A peculiar class of i + j rectangle is that of i + 6 rectangles in which entry is the score for ΔZi + Δ Z6 = . i.e. ΔZi = ., entered in a 31 x wi rectangle. In particular if i = 2 all entries lie on the principal diagonal and the rectangle degenerates into 
.
A rectangle which makes full use of the run 4 = 5./1 = 2 requires 4 entries (3 independent) in each cell (c.f. 25C(e) R1 p 62)
Several members of the section have contemplated "Rectangular parallelepipeds": probably the most favourable is i + j + 6/, ΔZ6 being always dot.
Rectangles may be combined, thus
|
||||||
|
|
|||||
but in practice this is done only for key (26C), because in cipher, pips in different rectangles are of unequal value.
(For Motor Rectangles see Appendix 92)
Back to General Report on Tunny. Contents.